NCERT Solutions for Class 11 Maths Chapter 3 Trigonometric Functions
Get Free NCERT Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.1, Ex 3.2, Ex 3.3, Ex 3.4, and Miscellaneous Exercise PDF in Hindi and English Medium. Trigonometric Functions Class 11 Maths NCERT Solutions are extremely helpful while doing your homework. Trigonometric Functions All Exercises Class 11 Maths NCERT Solutions
NCERT Solutions for Class 11 Maths Chapter 3 Trigonometric Functions
| Section Name | Topic Name |
| 3.1 | Introduction |
| 3.2 | Angles |
| 3.3 | Trigonometric Functions |
| 3.4 | Trigonometric Functions of Sum and Difference of Two Angles |
| 3.5 | Trigonometric Equations |
| 3.6 | Summary |
NCERT Solutions for Class 11 Maths Chapter 3 Exercise 3.1
Ex 3.1 Class 11 Maths Question 1:
Find the radian measures corresponding to the following degree measures:
(i) 25°
(ii) – 47° 30′
(iii) 240°
(iv) 520°
Ans:
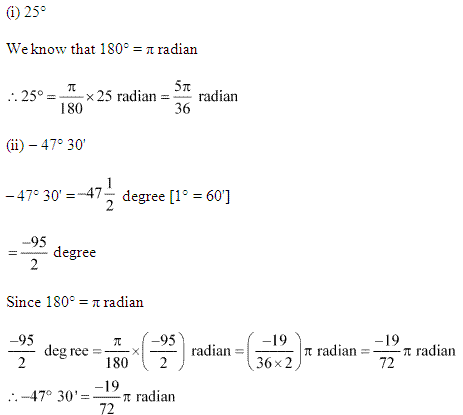


Ex 3.1 Class 11 Maths Question 2: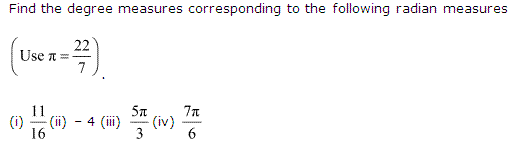
Ans:
(i)
We know that: π radian = 180°
∴
=
=
=
= 39
= 39° +
= 39° + 22′ +
= 39°22’30” [1′ = 60°].

Ex 3.1 Class 11 Maths Question 3:
A wheel makes 360 revolutions in one minute. Through how many radians does it turn in one second?
Ans:
Number of revolutions made by the wheel in 1 minute = 360
∴ Number of revolutions made by the wheel in 1 second =
In one complete revolution, the wheel turns an angle of 2π radian.
Hence, in 6 complete revolutions, it will turn an angle of 6 × 2π radian, i.e., 12π radian
Thus, in one second, the wheel turns an angle of 12π radian.
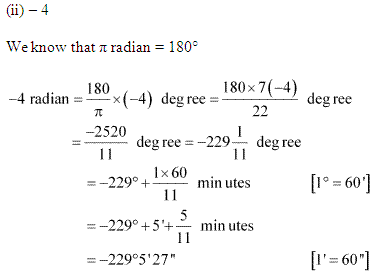
Ex 3.1 Class 11 Maths Question 4:
Find the degree measure of the angle subtended at the centre of a circle of radius 100 cm y an arc of length 22 cm (Use π =
Ans:
We know that in a circle of radius r unit, if an arc of length l unit subtends an angle θ radian at the centre, then θ =
Therefore, for r = 100 cm, l = 22 cm,
we have
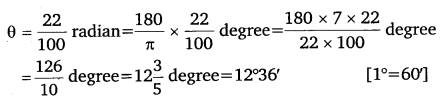
Thus, the required angle is 12°36′.
Ex 3.1 Class 11 Maths Question 5:
In a circle of diameter 40 cm, the length of a chord is 20 cm. Find the length of minor arc of the chord.
Ans:
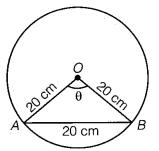
Given, diameter = 40 cm
∴ radius (r) =
and length of chord, AB = 20 cm
Thus, ∆OAB is an equilateral triangle.
We know that,
θ =
⇒ Arc AB = θ × r
=
=
Ex 3.1 Class 11 Maths Question 6:
If in two circles, arcs of the same length subtend angles 60° and 75° at the centre, find the ratio of their radii.
Ans:
Let the radii of the two circles be r1 and r2.
Let an arc of length l subtend an angle of 60° at the centre of the circle of radius r1, while let an arc of length l subtend an angle of 75° at the centre of the circle of radius r2.
Now, 6o° =
75° =
We know that in a circle of radius r unit, if an arc of length l unit subtends an angle θ radian at the centre, then θ =
∴ l =
l =
⇒
⇒ r =
Thus, the ratio of the radii is 5 : 4.
Ex 3.1 Class 11 Maths Question 7:
Find the angle in radian through which a pendulum swings if its length is 75 cm and the tip describes an arc of length
(i) 10 cm
(ii) 15 cm
(iii) 21 cm.
Ans:
We know that in a circle of radius r unit, if an arc of length l unit subtends an angle θ radian at the centre, then
θ =
It is given that r = 75 cm
(i) Here, l = 10 cm
θ =
=
(ii) Here, l = 15 cm
θ =
θ =
(iii) Here, l = 21 cm
θ =
=
NCERT Solutions for Class 11 Maths Chapter 3 Exercise 3.2
Ex 3.2 Class 11 Maths Question 1:Find the values of other five trigonometric functions if cos x = – 12 x lies in third quadrant.
Ans:
Ex 3.2 Class 11 Maths Question 1:
Find the values of other five trigonometric functions if cos x = –
Ans:
Ex 3.2 Class 11 Maths Question 2:
Find the values of other five trigonometric functions if sin x =
Ans:
sin x =
cosec x =
sin2 x + cos2 x = 1
⇒ cos2 x = 1 – sin2 x
⇒ cos2 x = 1 – (
⇒ cos2 x = 1 –
⇒ cos2 x =
⇒ cos x = ±
Since x lies in the 2nd quadrant, the value of cos x will be negative
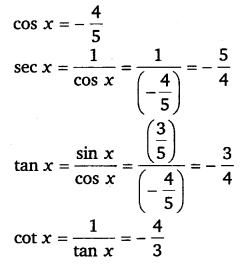
Ex 3.2 Class 11 Maths Question 3:
Find the values of other five trigonometric functions if cot x =
Ans: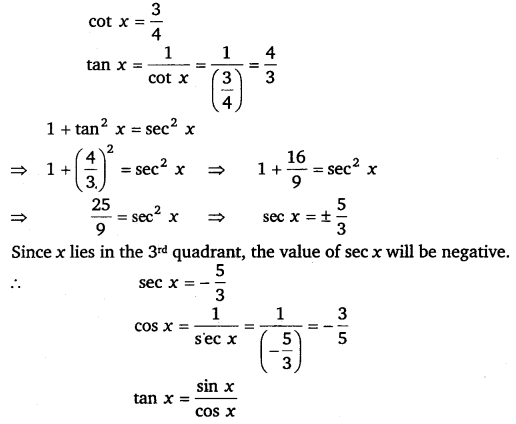
⇒
⇒ sin x =
⇒ cosec x =
Ex 3.2 Class 11 Maths Question 4:
Find the values of other five trigonometric functions if sec x =
Ans:
Ex 3.2 Class 11 Maths Question 5:
Find the values of other five trigonometric functions if tan x =
Ans:
tan x = –
cot x =
1 + tan2 x = sec2 x
Ex 3.2 Class 11 Maths Question 6:
Find the value of the trigonometric function sin 765°.
Ans:
It is known that the values of sin x repeat after an interval of 2π or 360°.
∴ sin 765° = sin (2 × 360° + 45°)
= sin 45° = 1
Ex 3.2 Class 11 Maths Question 7:
Find the value of the trigonometric function cosec (- 1410°)
Ans:
It is known that the values of cosec x repeat after an interval of 2π or 360°.
∴ cosec (- 1410°) = cosec (- 1410° + 4 x 360°)
= cosec (- 1410° + 1440°)
= cosec 30° = 2.
Ex 3.2 Class 11 Maths Question 8:
Find the value of the trigonometric function tan
Ans:
It is known that the values of tan x repeat after an interval of π or 180°.
∴
=
= tan 60° = √3.
Ex 3.2 Class 11 Maths Question 9:
Find the value of the trigonometric function sin
Ans:
It is known that the values of cot x repeat after an interval of π or 180°.
∴
=
Ex 3.2 Class 11 Maths Question 10:
Find the value of the trigonometric function cot
Ans:
It is known that the values of cot x repeat after an interval of ir or 1800.
∴
NCERT Solutions for Class 11 Maths Chapter 3 Exercise 3.3
Ex 3.3 Class 11 Maths Question 1:
Prove that: sin2
Ans:
L.H.S.= sin2
=
=
= R.H.S.
Hence proved.
Ex 3.3 Class 11 Maths Question 2:
Prove that: 2 sin2
Ans: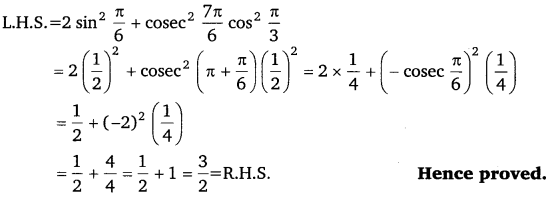
Ex 3.3 Class 11 Maths Question 3:
Prove that :cot2
Ans: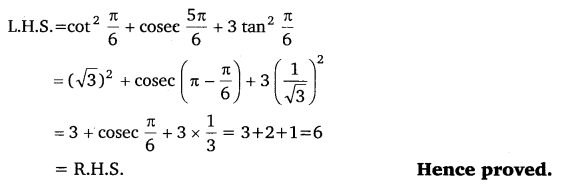
Ex 3.3 Class 11 Maths Question 4:
Prove that: 2 sin2
Ans:
L.H.S =
=
=
= 2
= 1 + 1 + 8
= 10 = R.H.S.
Hence proved.
Ex 3.3 Class 11 Maths Question 5:
Find the value of: (i) sin 75°,
(ii) tan 15°
Ans:
(i) sin 75° sin (45° + 30°)
= sin 45° cos 30° + cos 45° sin 30°
[∵ sin (x + y) = sin x cos y + cos x sin y]
=
=
(ii) tan 15° = tan (45° – 30°)
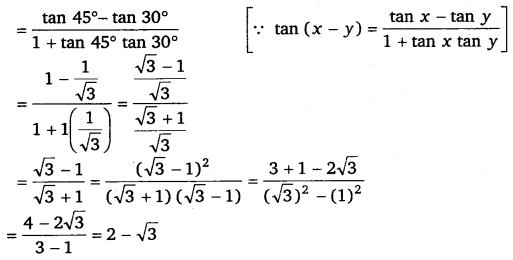
Ex 3.3 Class 11 Maths Question 6:
Ans: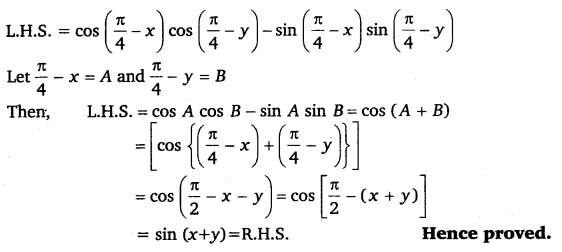
Ex 3.3 Class 11 Maths Question 7:
Prove that:
Ans: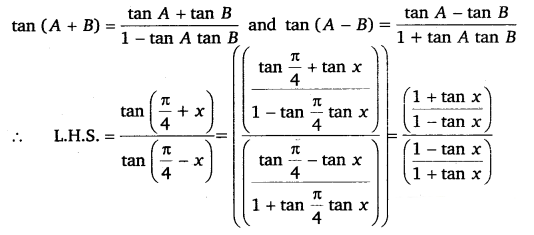
=
= R.H.S
Hence proved.
Ex 3.3 Class 11 Maths Question 8:
Prove that:
Ans:
L.H.S =
=
= cot2 x
= R.H.S
Hence proved.
Ex 3.3 Class 11 Maths Question 9: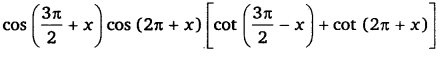 = 1
= 1
Ans: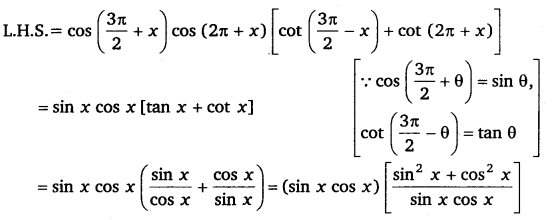
= 1 = R.H.S
Hence proved.
Ex 3.3 Class 11 Maths Question 10:
Prove that: sin (n + 1) x sin (n + 2) x + cos (n + 1) x cos (n + 2) x = cos x
Ans:
L.H. S. = sin (n + 1 )x sin (n + 2) x + cos (n +1) x cos (n + 2) x
[By the formula, cos (A – B) = cos A cos B + sin A sin B]
= cos [(n + 2) x + (n + 1) x]
= cos (4x + 2x – 4x – x)
= cos x = R.H.S.
Hence proved.
Ex 3.3 Class 11 Maths Question 11:
Prove that:
Ans:
It is known that
cos A – cos B =
∴ L.H.S.=
=
= – 2 sin (
= – 2 sin (-
= – √2 sin x = R.H.S.
Hence proved.
Ex 3.3 Class 11 Maths Question 12:
Prove that: sin2 6x – sin2 4x = sin 2x sin 10 x
Ans:
It is known that
sin A + sin B = 2
sin A – sin B = 2
L.H.S.= sin2 6x – sin2 4x
= (sin 6x + sin 4x) (sin 6x – sin 4x)
= (2 sin 5x cos x) (2 cos 5x sin x)
= (2 sin 5x cos 5x) (2 sin x cos x)
= sin 10x sin 2x = R.H.S.
Hence proved.
Ex 3.3 Class 11 Maths Question 13:
Prove that: cos2 2x cos2 6x = sin 4x sin 8x
Ans:
It is known that
cos A + cos B = 2
cos A – cos = 2
∴ L.H.S = cos2 2x – cos2 6x
= (cos 2x + cos 6x) (cos 2x – 6x)
=
∴ L.H.S.= cos2 2x – cos2 6x
= (cos 2x + cos 6x) (cos 2x – 6x)
= [2 cos 4x cos (-2x)] [- 2 sin 4x sin (- 2x)]
= [2 cos 4x cos 2x] [- 2 sin 4x (- sin 2x)]
= (2 sin 4x cos 4x) (2 sin 2x cos 2x)
= sin 8x sin 4x
= R.H.S.
Hence proved.
Ex 3.3 Class 11 Maths Question 14:
Prove that: sin 2x + 2 sin 4x + sin 6x = 4 cos2 x sin 4x
Ans:
L.H.S.= sin 2x + 2 sin 4x + sin 6x
= [sin 2x + sin 6x] + 2 sin 4x
=
[∵ sin A + sin B = 2
= 2 sin 4x cos (- 2x) + 2 sin 4x
= 2 sin 4x cos 2x + 2 sin 4x
= 2 sin 4x (cos 2x + 1)
= 2 sin 4x (2 cos2 x – 1 + 1)
= 2 sin 4x (2 cos2 x)
= 4 cos2 x sin 4x
= R.H.S.
Hence proved.
Ex 3.3 Class 11 Maths Question 15:
cot 4x (sin 5x + sin 3x) = cot x (sin 5x – sin 3x)
Ans: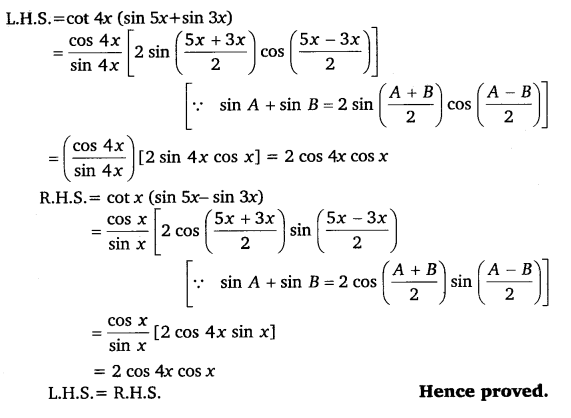
Ex 3.3 Class 11 Maths Question 16:
Prove that:
Ans: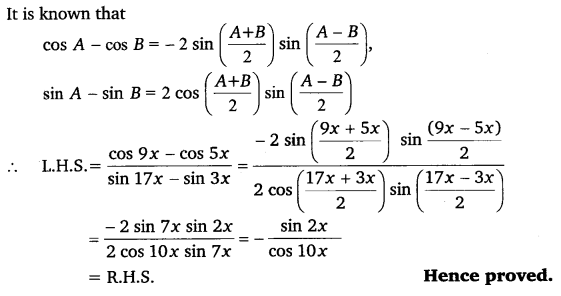
Ex 3.3 Class 11 Maths Question 17:
Prove that:
Ans:
It is known that
sin A + sin = 2
cos A + cos = 2
∴ L.H.S =
=
=
= tan 4x = R.H.S.
Hence proved.
Ex 3.3 Class 11 Maths Question 18:
Prove that:
Ans: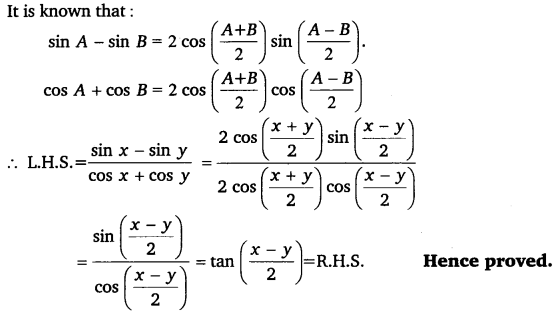
Ex 3.3 Class 11 Maths Question 19:
Prove that:
Ans: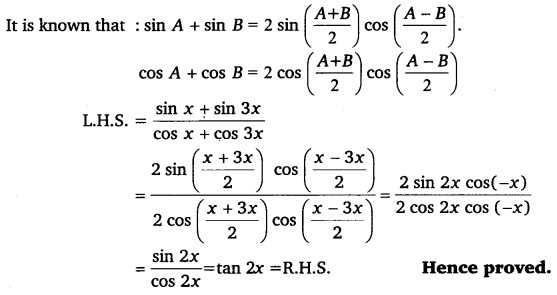
Ex 3.3 Class 11 Maths Question 20:
Prove that:
Ans:
It is known that
sin A – sin B = 2
cos2 A – sin2 A = cos 2A
∴ L.H.S. =
=
=
= – 2 × (- sin x) = 2 sin x
= R.H.S
Hence proved.
Ex 3.3 Class 11 Maths Question 21:
Prove that:
Ans: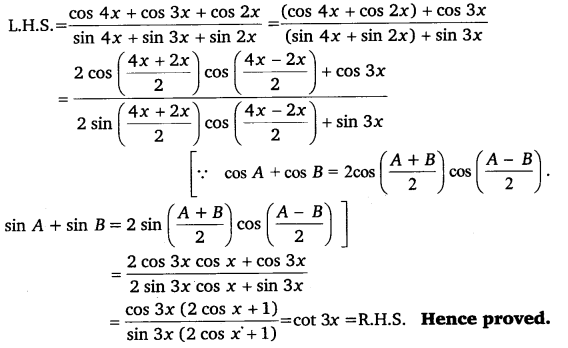
Ex 3.3 Class 11 Maths Question 22:
Prove that : cot x cot 2x – cot 2x cot 3x – cot 3x cot x = 1
Ans:
L.H.S.= cot x cot 2x – cot 2x cot 3x – cot 3x cot x
= cot x cot 2x – cot 3x (cot 2x + cot x)
= cot x cot 2x – cot (2x + x) (cot 2x + cot x)
= cot x cot 2x –
[∵ cot (A + B) =
= cot x cot 2x – (cot 2x cot x – 1)
= 1 = R.H.S.
Hence proved.
Ex 3.3 Class 11 Maths Question 23:
Prove that: tan 4x =
Ans:
It is known that:
tan 2A = 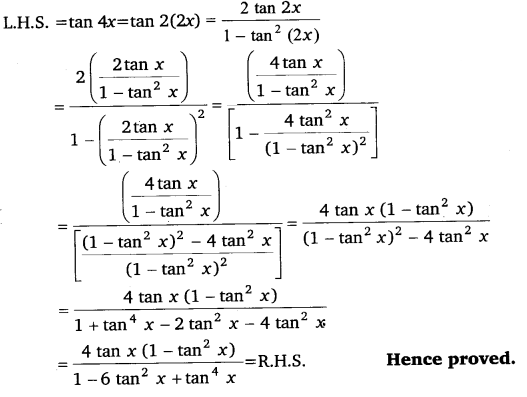
Ex 3.3 Class 11 Maths Question 24:
Prove that: cos 4x = 18 sin2 x cos2 x
Ans:
L.H.S. = cos 4x = cos 2(2x)
= 1 – 2 sin2 2x [∵ cos 2A = 1 – 2 sin2 A]
= 1 – 2(2 sin x cos x)2 [∵ sin 2A = 2 sin A cos A]
= 1 – 8 sin2 x cos2 x
= R.H.S.
Hence proved.
Ex 3.3 Class 11 Maths Question 25:
Prove that: cos 6x = 32 cos6 x – 48 cos4 x + 18 cos2 x – 1
Ans:
We know that: cos 3x = 4 cos3 x – 3cos x
On replacing x by 2x, we get
cos 3(2x) = 4 cos3 (2x) – 3 cos 2x
⇒ cos 6x = 4 (2cos2 x – 1)3 – 3 (2cos2 x – 1)
[∵ cos 2x = 2cos2 x – 1]
= 4 [8 cos6 x – 12 cos4 x + 6 cos2 x – 1] – 6 cos2 x + 3
[∵ (a – b)3 = a3 – 3a2b + 3ab2 – b3]
= 32 cos6 x – 48 cos4 x + 24 cos2 x – 4 – 6 cos2 x + 3
⇒ cos 6x = 32 cos6 x – 48 cos4 x + 18 cos2 x – 1
Hence proved.
Class 11 Maths NCERT Solutions Chapter 3 Exercise 3.4
Ex 3.4 Class 11 Maths Question 1:
Find the principal and general solutions of the equation, tan x = √3
Ans:
tan x = √3
It is known that:
tan
tan (
= tan
Therefore, the principal solutions are x =
Now, tan x = tan
⇒ x = nπ +
Therefore, the general solution is x = nπ +
Ex 3.4 Class 11 Maths Question 2:
Find the principal and general solutions of the equation: sec x = 2
Ans:
sec x = 2
It is known that:
sec
sec
= sec
Therefore, the principal solutions are x =
Now, sec x = sec
cos x = cos
⇒ x = 2nπ ±
Therefore, the general solution is x = 2nπ ±
Ex 3.4 Class 11 Maths Question 3:
Find the principal and general solutions of the equation cot cot x = – √3.
Ans:
cot x = – √3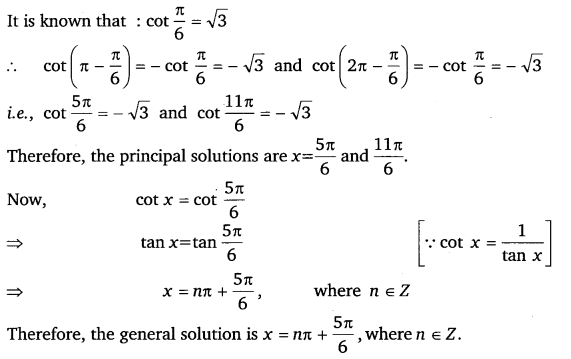
Ex 3.4 Class 11 Maths Question 4:
Find the principal and general solutions of cosec x = – 2
Ans:
cosec x = – 2
It is known that:
cosec 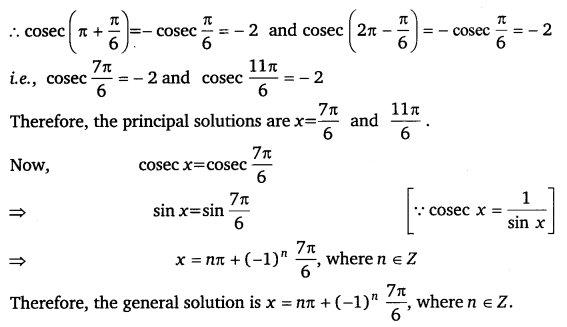
Ex 3.4 Class 11 Maths Question 5:
Find the general solution of the equation: cos 4x = cos 2x
Ans:
cos 4x = cos 2x
cos 4x – cos 2x = 0
– 2 sin
[∵ cos A – cos B = 2 \sin \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)
sin 3x sin x = 0
sin 3x = 0or sin x = 0
3x = nπ or x = nπ, where n ∈ Z
x =
Ex 3.4 Class 11 Maths Question 6:
Find the general solution of the equation cos 3x + cosx – cos 2x = 0
Ans:
cos 3x + cos x – cos 2x = 0
2 cos
[∵ cos A + cos B = 2
2 cos 2x cos x – cos 2x = 0
cos 2x (2 cos x – 1) = 0
cos 2x = 0 or 2 cos x – 1 = 0
cos 2x = 0 or cos x = \(\frac{1{2}\)
∴ 2x = (2n + 1)
x = (2n + 1)
Ex 3.4 Class 11 Maths Question 7:
Find the general solution of the equation sin 2x + cos x = 0
Ans:
sin 2x + cos x = 0
⇒ 2sin x cos x + cos x = 0
⇒ cos x (2 sin x + 1) = 0
⇒ cos x = 0 or 2 sin x + 1 = 0
Now, cos x = 0
⇒ x = (2n + 1)
or 2 sin x + 1 = 0
⇒ sin x = –
= – sin
= sin (π +
= sin
x = nπ + (- 1)n
Therefore, the general solution is (2n + 1)
Ex 3.4 Class 11 Maths Question 8:
Find the general solution of the equation sec2 2x = 1 – tan 2x.
Ans:
sec2 2x = 1 – tan 2x
1 + tan2 2x = 1 – tan 2x
tan2 x + tan 2x = 0
=> tan 2x (tan 2x + 1) = 0
=> tan 2x = 0 or tan 2x + 1 = 0
Now, tan 2x = 0
=> tan 2x = tan 0
2x = nπ + 0, where n ∈ Z
x =
or tan 2x + 1 = 0
= tan 2x = – 1
= – tan
= tan (π –
= tan
2x = nπ +
x =
Therefore, the general solution is
Ex 3.4 Class 11 Maths Question 9:
Find the general solution of the equation sin x + sin 3x + sin 5x = 0
Ans:
sin x + sin 3x + sin 5x = 0
⇒ (sin x + sin 5x) + sin 3x = 0
[∵ sin A + sin B = 2 sin
2 sin 3x cos (2x) + sin 3x = 0
2 sin 3x cos 2x + sin 3x = 0
sin 3x (2 cos 2x +1) = 0
sin 3x = 0 or 2 cos 2x + 1 = 0
Now sin 3x = 0
⇒ 3x = nπ, where n ∈ Z
i.e., x =
or 2 cos 2x + 1 = 0
cos 2x =
= – cos
= cos (π –
cos 2x = cos
⇒ 2x = 2nπ ±
⇒ x = nπ ±
Therefore, the general solution is
NCERT Solutions for Class 11 Maths Chapter 3 Miscellaneous Exercise
Miscellaneous Exercise Class 11 Maths Question 1:
Prove that:
Ans: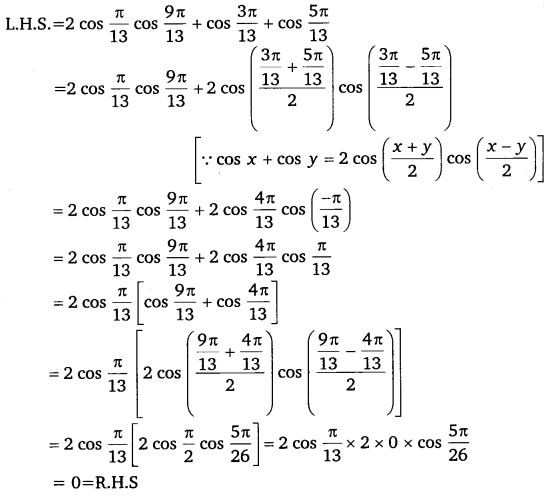
Hence proved.
Miscellaneous Exercise Class 11 Maths Question 2:
Prove that: (sin 3x + sin x) sin x + (cos 3x – cos x) cos x = 0.
Ans:
L.H.S. = (sin 3x + sin x) sin x + (cos 3x – cos x) cos x
= sin 3x sin x + sin2 x + cos 3x cos x – cos2 x
= cos 3x cos x + sin 3x sin x – (cos2 x – sin2 x)
= cos (3x – x) – cos 2x
[∵ cos(A – B) = cos A cos B + sin A sin B]
= cos 2x – cos 2x = 0
=R.H.S.
Hence proved.
Miscellaneous Exercise Class 11 Maths Question 3:
Prove that:
(cos x + cos y)2 + (sin x – sin y)2 = 4 cos2
Ans:
L.H.S.= (cos x + cos y)2 + (sin x – sin y)2
= cos2 x + cos2 y + 2 cos x cos y + sin2 x + sin2 y – 2 sin x sin y
= (cos2 x + sin2 x) + (cos2 y + sin2 y) + 2 (cos x cos y – sin x sin y)
= 1 + 1 + 2 cos (x + y)
[∵ cos (A + B) = (cos A cos B – sin A sin B)]
= 2 + 2 cos (x + y)
= 2 [1 + cos (x + y)]
= 2[1 +
[∵ cos 2A = 2 cos2 A – 1]
= 4 c0s2
= R.H.S.
Hence proved.
Miscellaneous Exercise Class 11 Maths Question 4:
Prove that:
(cos x – cos y)2 + (sin x – sin y)2 = 4 sin2
Ans:
L.H.S.= (cos x – cos y)2 + (sin x – sin y)2
= cos2 x + cos2 y – 2 cos x cos y + sin2 x + sin2 y – 2 sin x sin y
= (cos2 x + sin2 x) + (cos2 y + sin2 y) – 2 [cos x cos y + sin x sin y]
= 1 + 1 – 2 [cos (x – y)]
= 2 [1 – {1 – 2 sin2
[∵ cos 2A = 1 – 2 sin2 A]
= 4 sin2
= R.H.S.
Hence proved.
Miscellaneous Exercise Class 11 Maths Question 5:
Prove that: sin x + sin 3x + sin 5x + sin 7x = 4 cos x cos 2x sin 4x
Ans:
It is known that sin A + sin B = 2
∴ L.H.S. = (sin x + sin 3x) + (sin 5x + sin 7x)
= (sin x + sin 5x) + (sin 3x + sin 7x)
=
= 2 sin 3x cos (- 2x) + 2 sin 5x cos (- 2x)
= 2 sin 3x cos 2x + 2 sin 5x cos 2x
= 2 cos 2x [sin 3x + sin 5x]
= 2 cos 2x [latex]2 \sin \left(\frac{3 x+5 x}{2}\right) \cdot \cos \left(\frac{3 x-5 x}{2}\right)[/latex]
= 2 cos 2x [2 sin 4x . cos (- x)]
= 4 cos 2x sin 4x cos x
= R.H.S.
Hence proved.
Miscellaneous Exercise Class 11 Maths Question 6:
Prove that:
Ans:
It is known that

Hence proved.
Miscellaneous Exercise Class 11 Maths Question 7:
Prove that: sin 3x + sin 2x – sin x = 4 sin x cos
Ans:
L.H.S. = sin 3x + sin 2x – sin x
= sin 3x + (sin 2x – sin x)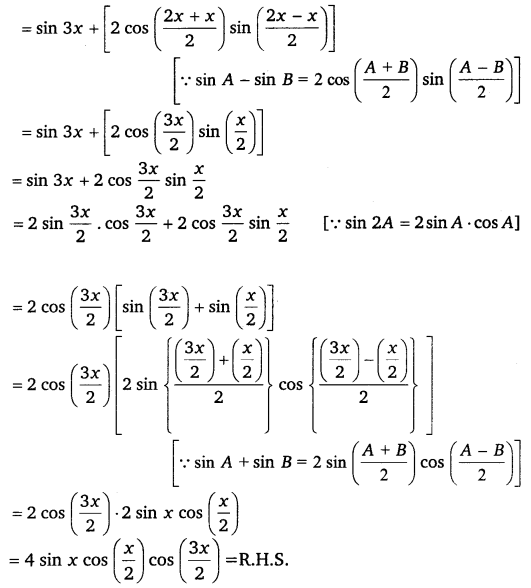
Miscellaneous Exercise Class 11 Maths Question 8:
Find sin
Ans: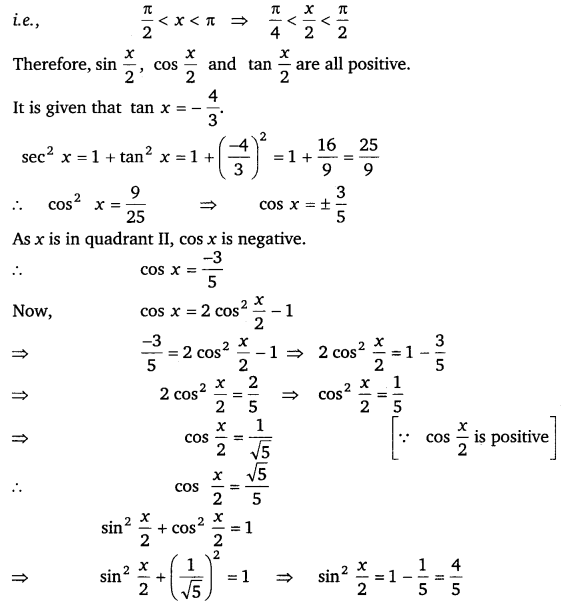
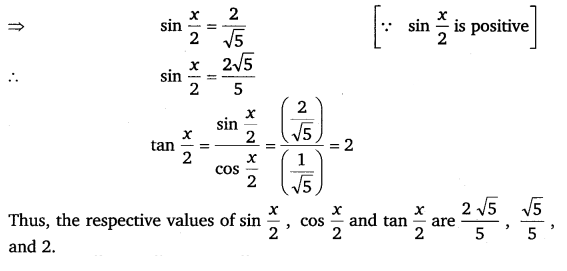
Miscellaneous Exercise Class 11 Maths Question 9:
Find sin
Ans: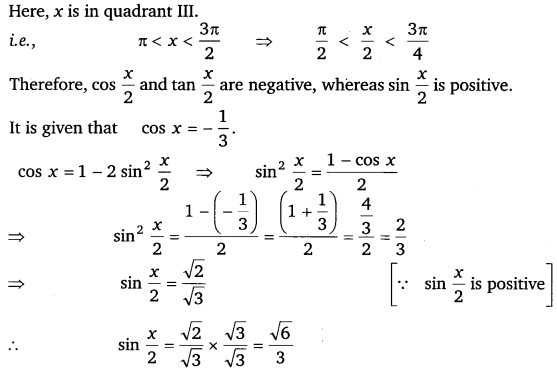
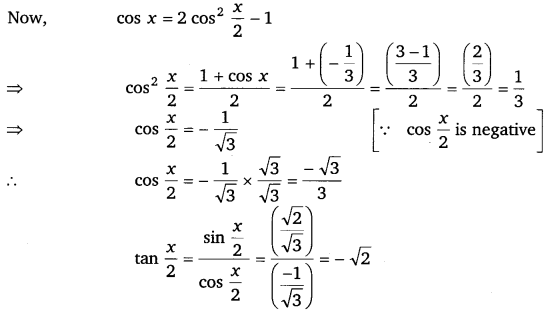
Thus, the respective values of sin
Miscellaneous Exercise Class 11 Maths Question 10:
Find sin
Ans: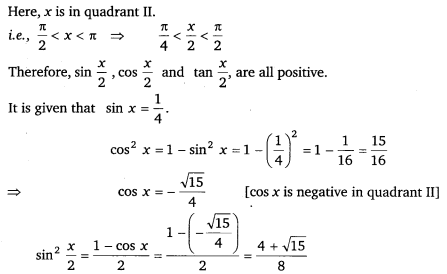
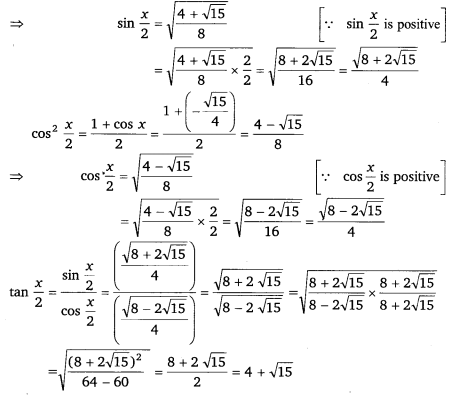
Thus, the respective values of sin







